The Meaning of Slutsky

Business cycles, and their rhythms, have long fascinated and perplexed economists. Why do economic booms alternate with recessions, decade after decade? And why do graphs of long-term data on gross domestic product, employment and other economic indicators form undulating patterns similar to physical phenomena such as ocean waves or sound waves? Over the past 150 years, all sorts of explanations have been put forth for recurrent peaks and valleys in economic activity—economists have hypothesized forces as seemingly far-fetched as sunspot activity and rainfall patterns as the cause of these cyclical patterns in national and world economies.

By the early 20th century, some researchers believed that chance occurrences like wars, crop failures and technological innovations played a role in business cycles. But no one fully appreciated how crucial random (or “stochastic”) processes are to the workings of the economy until Eugen Slutsky, a Soviet statistician and econometrician, did the math. A middle-aged professor working at a Moscow think tank, Slutsky was virtually unknown to economists in Europe and the United States when he published his landmark paper on cyclical phenomena in 1927.¹

In a bold statistical experiment, Slutsky demonstrated that random numbers subjected to statistical calculations similar to those used to reveal trends in economic time-series formed wavelike patterns indistinguishable from business cycles. The implication was that a similar stochastic process—“the summation of random causes,” as Slutsky described it—might be at work in the actual economy, causing prosperity to ebb and flow without the agency of sunspots, meteorological patterns or other cyclical forces.

“That was a hell of an idea,” said Robert Lucas, a University of Chicago economist who pioneered modern business cycle theory, in an interview. “It was just a huge jump from what anyone had done.”

Today, Slutsky is more familiar among economists for his earlier work in consumer theory. Every economics undergraduate learns the Slutsky equation, which analyzes shifts in demand for goods by looking at two components, the income and substitution effects of price changes (see The Mechanics of Demand). But Slutsky’s 1927 paper made an enormous contribution to business cycle theory that forever changed the way economists view economic fluctuations.

Following a peak during the Great Depression, interest in divining the causes of booms and recessions waned after World War II. But with further economic turmoil in the 1970s and 1980s, researchers again became fascinated with business cycles—and with the role of shocks (both random and nonrandom) in propagating them. Slutsky’s enduring insight, combined with advances in economic growth theory, gave rise to modern macroeconomic models that simulate the impact of shocks such as new technologies, energy price hikes, changes in consumer preferences and tax increases or cuts.

Much of the work over the past 25 years on “real business cycles”—the idea that economic oscillations stem from “real” shocks such as innovations or changes in regulations rather than “nonreal” factors such as price trends, interest rates and monetary policy—has been done by investigators associated with the Federal Reserve Bank of Minneapolis. For example, Edward Prescott, a monetary adviser to the Fed, has proposed random shocks to productivity as a key driver of fluctuations from the constant growth trend in U.S. GDP.

However, economists continue to grapple with basic questions about random shocks and their impact on the economy. What are these shocks, exactly, and how do they interact with labor productivity, capital investment, fiscal policy and other factors to cause economic expansions and contractions?

If Slutsky’s energies had remained focused on economics, he might have investigated those questions himself. Instead, he chose to work in statistics and mathematics for the rest of his career—a switch possibly motivated by fears for his life. Joseph Stalin took a dim view of theories that didn’t fit his framework for a centrally planned economy.

When Slutsky died in 1948, he probably had no inkling of the lasting impact his experiment with random numbers would have on macroeconomics. His work sparked a crucial intuition about market economies: Stuff happens. The economy is a dynamic entity that reacts unpredictably to random events (at least in the short term) and resists efforts to smooth out bumps in the road.

Making sense of cycles

Knut Wicksell, in the early 1900s, was perhaps the first economist to suggest that random shocks are complicit in the boom-bust cycles characteristic of market economies. Theorizing that erratic, unforeseen events such as innovations provide much of the impetus behind business cycles, he drew a simple analogy: “If you hit a wooden rocking-horse with a club, the movement of the horse will be very different to that of the club.”² That is, irregular blows to the rocking-horse will make it swing in a more or less regular arc.

In the 1920s, fellow Swede Johan Åkerman elaborated on this idea, comparing random economic shocks to pebbles on a streambed; such irregularities generate regular waves on the stream’s surface.

These images of regularity arising from randomness were at odds with prevailing theories that ascribed business cycles to some underlying, often hidden force. U.S. economist Henry Moore postulated an eight-year meteorological cycle that drove fluctuations in harvests and the production of raw materials. Another American economist, Wesley Mitchell, broke down business cycles into periods of prosperity, crisis, depression and revival in which each phase created the conditions for the next. This metronomic view of the economy was taught at many U.S. universities in the 1920s and 1930s.

Though Wicksell and Åkerman raised the possibility that stochastic processes were involved in business cycles, nobody had demonstrated the mechanism by which random events could cause recurrent, fairly regular oscillations in economic activity. That task fell to Slutsky, a researcher at the Moscow Conjuncture Institute, a government-run organization devoted to the study of business conditions in the young Soviet Union.

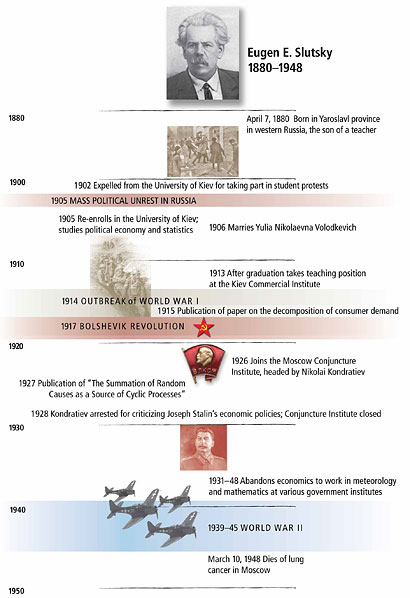
In 1927, Slutsky was a 47-year-old math and statistics whiz who had traveled a long road to attain his position among the country’s intellectual elite. In his youth, he had taken part in student protests against Czarist policies; expelled twice from the University of Kiev and barred from attending university anywhere within the Russian Empire, he was forced to leave the country to continue his education. Studying engineering in Germany, Slutsky developed instead a keen interest in economics that blossomed when he was allowed to re-enroll at the University of Kiev in 1905. “I already had plans for working on the application of mathematics to economics,” he wrote decades later in an autobiographical sketch.³

As he studied political economy, he became enthralled with the new statistical techniques of British mathematician Karl Pearson and wrote a well-received book on mathematical statistics. The book was published in 1912, a year after Slutsky finally got his bachelor’s degree at the ripe age of 31. Slutsky’s famous paper on consumer demand followed in 1915, after he had secured a teaching post at the Kiev Commercial Institute.

By the mid-1920s, Slutsky was working on probability theory and a variety of economic topics steeped in mathematics and statistics. Slutsky hints in his autobiography that his decision to focus on the technical side of economics was motivated at least in part by political events in the wake of the Bolshevik Revolution—the fall of capitalism and its replacement by a state-controlled economy.

In 1926, Slutsky landed a plum post at the Conjuncture Institute in Moscow, headed by renowned Soviet economist Nikolai Kondratiev. There he threw himself into an intense study of apparent cycles in economic time-series. He was familiar with the theories of Moore, Mitchell and his boss Kondratiev, who posited “long-wave” business cycles of 50 to 60 years in market economies. But he saw another potential driver of business cycles, one hinted at by British mathematician George Udny Yule’s recent work on “nonsense correlations” in economic data. Could the laws of probability account for the recurrent spikes and dips seen in time-series? To find out, Slutsky performed statistical experiments on random numbers from a government lottery.

He wrote excitedly to his wife Yulia in Kiev that he was “lucky to arrive at a rather considerable finding, to discover the secret of … those wavy movements that are observed in social phenomena.”⁴

Luck of the draw

Slutsky’s method was unorthodox at the time—indeed, it was revolutionary. Instead of coming up with a business cycle theory and then using it to try to explain observed historical data, he manipulated an artificial set of data to see what patterns emerged. His approach was inductive and agnostic, noted Lucas. It didn’t start from an initial belief or hypothesis, but rather arrived at a theory after examining mathematical facts. “There’s no particular view of business cycles advanced in the paper,” said Lucas. “What he shows is that if you construct a simulated time-series … you can generate patterns that look just like the patterns we see in economic time-series.”

The statistical model that Slutsky created was a forerunner of modern computer simulations of random systems, the “Monte Carlo” methods used today in fields ranging from economics and finance to engineering and meteorology.

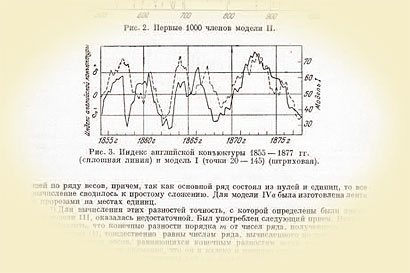
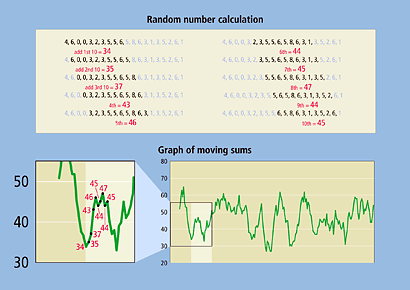
Assisted by Institute staffers armed with sharp pencils, Slutsky took random lottery numbers and added them sequentially. He created a new series of numbers consisting of the sum of a given random digit plus the nine that preceded it, then the sum of the next digit plus the previous nine, then the sum of the next digit plus … and so on. The process is akin to repeatedly rolling dice and adding up the values of the previous rolls; in Slutsky’s case, he calculated a 10-item moving sum of random digits, producing a new numerical series analogous to the dice totals (see In Sum: Slutsky's Experiment below, upper panel).

In Sum: Slutsky's Experiment

Slutsky’s simplest model began with a long string of random numbers. A 10-item moving sum of these produced a second series (34,35,37 and so on in this example). A graph of this series formed wave patterns similar to time-series economic data. Slutsky showed that output from his random-number model bore a striking resemblance to actual British business cycles.

The method is similar to that used to track stock prices and currency exchange rates, where computers calculate moving averages (rather than sums) to smooth the jagged profile of hourly or daily observations, letting analysts discern broad trends over time.

The key to Slutsky’s model is that the moving summation process forges connections among the numbers derived from the original series of completely random lottery numbers. In statistical terms, they become serially correlated; the value of each sum is associated with, but not identical to, the values of previous sums because they share nine out of 10 elements. This means that the effect of a single random number persists in the chain of moving summations. If that digit comes up several times in close succession—as can occur in any random drawing—it can skew the moving sums either high or low. Over time, this process causes the values of the sums to oscillate—the statistical equivalent of a seesaw.

When Slutsky and assistants finished their tedious calculations, the result was breathtaking—to a statistician at least. Their series of sums of random numbers created a nonrandom pattern. When graphed, it described a wave-like curve similar to cycles in time-series of aggregate output, employment and other economic variables. One section of Slutsky’s moving-summation plot closely matched an index of British business cycles from 1855 to 1877 (see In Sum: Slutsky's Experiment above, bottom panel), “an initial graphic demonstration of the possible effects of the summation of unconnected causes,” he wrote.⁵

Other models in the paper that use more complex moving summations generated similar cyclical patterns—all derived from numbers pulled purely at random. (Slutsky would have seen similar patterns if he had summed dice rolls; charts of such series bear an uncanny resemblance to fluctuations in stock prices.)

Slutsky’s discovery—that the moving summation or average of a random series may generate oscillations when no such movements exist in the original data—is called the Slutsky-Yule effect. (Yule, in a 1927 paper, arrived independently at the same finding.) Slutsky later proved that when the number of summations approaches infinity, the randomly generated undulations form sine waves—smooth arcs like ocean swells, or the pulse of alternating electric current.

Slutsky had shown in dramatic fashion that stochastic processes could create patterns virtually identical to the putative effects of weather patterns, self-perpetuating boom-bust phases and other factors on the economy. The obvious question was whether—as Slutsky’s paper seemed to imply—series of random events actually contributed to business cycles in the real world.

Deconstructing Slutsky

“The Summation of Random Causes as a Source of Cyclic Processes” was written in Russian; the paper wasn’t widely available to Western economists until 10 years later, when a longer English version was published in the journal Econometrica. But the few business cycle researchers in Europe and the United States who gained access to Slutsky’s 1927 paper immediately recognized its significance. A random element had to be accounted for in analyzing economic time-series.

One interpretation of Slutsky’s work is that the remarkable synchrony he found between simulated cycles and the ups and downs of the British economy is an artifact of statistics, an illusion with no bearing on the movements of the real economy. But Western economists were intrigued by an alternative reading of his paper: The similarity of randomly generated cycles to actual business cycles is no accident; random events such as inventions, storms and conflicts somehow shape the rhythms of the real-life economy. (Slutsky himself isn’t clear on the matter, although the paper’s title and the letter to his wife suggest that he favored this view.)

Simon Kuznets, a Russian émigré to the United States who studied under Mitchell at Columbia University, reviewed Slutsky’s findings and conducted statistical experiments on time-series to test his ideas. In a 1929 paper, Kuznets theorized that a careful analysis of business cycles would reveal the signatures of different types of random shocks acting upon the economy: a string of small shocks versus one or two big shocks, for example. He also noted the ramifications of this line of reasoning for the business cycle theories of Mitchell and his contemporaries: “If cycles arise from random events … then we obviously do not need the hypothesis of an independent regularly recurring cause which is deemed necessary by some theorists of business cycles.”⁶

Norwegian economist Ragnar Frisch also seized upon Slutsky’s findings in his 1933 analysis of the forces driving business cycles. Hitching Slutsky’s work to Wicksell’s rocking-horse analogy, Frisch (co-winner of the first Nobel Prize in economics) developed a dynamic macroeconomic model that incorporated random shocks.

In his model, delays in capital investment needed to satisfy increased consumer demand cause recurrent oscillations in economic output—the swings of the rocking-horse. But the rocking-horse would come to rest after two or three cycles without some external force acting upon it. Frisch wondered what would happen if the horse were hit with a club—“a stream of erratic shocks that constantly upsets the continuous evolution, and by so doing introduces into the system the energy necessary to maintain the swings.”⁷ He mimicked such shocks with a “stochastic difference equation”—a mathematical apparatus still used today to simulate the impact of chance events on economies.

While Western economists were pondering the meaning of Slutsky, the man himself had abandoned economics to apply his statistical acumen to hydrology and meteorology. In 1928, Stalin had released a five-year master plan for controlling every aspect of the Soviet economy. When Kondratiev dared to criticize the plan, the Conjuncture Institute was shut down, and its former director imprisoned and later executed. Slutsky realized that continuing to work in economics—even on abstruse theoretical topics—was too dangerous under Stalin’s rule, said John Chipman, an economics professor at the University of Minnesota who has studied Slutsky’s career.

“He saw what happened to Kondratiev,” Chipman said in an interview. “I think it’s incontrovertible that Slutsky switched fields in order to preserve his life.” Tellingly, in his 1938 autobiography, written as part of a job application, Slutsky skips over his two-year tenure at the Conjuncture Institute.

In the 1930s and during World War II, working in government research posts, Slutsky studied weather patterns instead of business cycles. In his last years, he performed important but laborious duty in statistics, preparing tables of probabilities for various distribution functions. When he died at age 67 of lung cancer, his obituary was written by the great Soviet mathematician Andrey Kolmogorov.

Business cycles revisited

After World War II, economists largely lost interest in business cycles. In an era of rising global prosperity, the emphasis was on measuring economic growth and fine-tuning it by applying Keynesian stabilization policy. In the United States, the Cowles Commission for Research in Economics developed complex macroeconomic models designed to identify optimum levels of government spending and taxation to achieve economic growth and full employment. In the 1960s, economic advisers to the Kennedy administration shaped tax and spending policies in an ill-fated effort to eliminate recessions altogether.

“There was a period when people thought that business cycles no longer exist, and we don’t have to worry about them in the future,” said Chipman, who was a researcher for the Cowles Commission in the 1950s.

But in the 1970s and 1980s, a new generation of economists—motivated in part by the “stagflation” of the 1970s, which showed that high inflation could coexist with high unemployment—looked at business cycles with fresh eyes. Lucas, Prescott and other investigators rediscovered the meaning of Slutsky and, blending his ideas with advances in modeling how economies grow, developed their own conceptions of rhythmic forces at work in the economy.

By the 1970s, many theorists had come to view the economy as a dynamic system that achieves a balance between the output of firms and household demand, even as its aggregate output fluctuates from quarter to quarter and year to year. In a 1977 article, Lucas defined the U.S. business cycle as “movements about trend in gross national product.”⁸ Over time, the economy grows at a fairly steady rate, but GDP oscillates around that trend, like a sailboat tacking back and forth in order to reach its destination. A series of positive deviations from trend rising to a peak constitutes an economic expansion, while a string of negative deviations leading to a trough indicates a recession. Like gusts buffeting the sailboat, these economic fluctuations are irregular and unpredictable.

Random shocks in dynamic models

A few years later Prescott and Finn Kydland, an economist at Carnegie Mellon University, proposed random events as the major motive behind these oscillations. In a seminal 1982 paper, Prescott (then at the University of Minnesota) and Kydland estimated that random shocks to productivity accounted for 70 percent of the fluctuations in U.S. economic growth after World War II. Their research was inspired in part by the findings of Slutsky and Frisch half a century earlier; in a later paper, Kydland and Prescott describe the work of both men.

Kydland and Prescott envisioned the effects of random shocks to productivity—new technology, energy price spikes, regulatory changes—accumulating over time. The reverberations of economic shocks linger for months or years, reinforcing the effects of new shocks and causing deviations from the long-term growth trend. Thus, the economy steers a serpentine course through booms and recessions.

To simulate these movements, Kydland and Prescott created a macroeconomic model in which households and firms optimally respond to changes in productivity, choosing to work and invest more or less when random shocks either increase or diminish the value of their labor and capital. The economists found that their model with random shocks, mirroring Slutsky’s results, produced estimates of fluctuations in GDP and other variables that corresponded with those in actual economic time-series—in this case, data on U.S. economic performance between 1950 and 1979.

By incorporating random shocks into a dynamic model in which individual agents act on their preferences, Kydland and Prescott, with Lucas and others, ushered in a revolution in macroeconomic theory. Today, dynamic stochastic general equilibrium (DSGE) models are standard tools for investigating business cycles and other macroeconomic phenomena. At the Minneapolis Fed, scholars have built upon Kydland and Prescott’s foundational work in real business cycles by modeling the impact of other types of nonmonetary shocks. For example, Ellen McGrattan, a University of Minnesota professor and monetary adviser to the Minneapolis Fed, has examined the impact of fiscal shocks such as tax changes on economic activity.⁹

Look out—shocks ahead

Precisely how random events exert their influence on the economy is still not fully understood. Researchers beg to differ on many aspects of the mechanics of business cycles, airing their opposing views in professional journals. Real business cycle theories, for example, have come under fire from economists who believe that shocks to consumer demand, or to the money supply, offer a better explanation for economic fluctuations.

One prominent example is Lawrence Summers, now director of the White House’s National Economic Council, who in 1986 criticized Prescott for, among other things, assuming that random shocks to productivity are at the root of economic fluctuations. “He provides no discussion of the source or nature of these shocks, nor does he cite any microeconomic evidence for their importance,” Summers wrote.¹⁰ Prescott offered a spirited reply.¹¹

Summers’ critique highlights a lack of consensus on the nature of the shocks constantly peppering the economy. Economists continue to posit all kinds of shocks as propagators of business cycles, but for the most part, their modeling efforts have focused not on the shocks, but on how the economy reacts to those shocks. To return to Wicksell’s analogy, more attention has been paid to the rocking-horse than to the club.

The deep recession of the past two years has raised anew questions about the interaction of chance events with government action (or inaction) in causing severe economic downturns. (Researcher interest in business cycle theory, it seems, follows a nonrandom, countercyclical pattern.) Some economists contend that misguided industrial regulation or monetary policy can exacerbate contractions already under way due to random shocks.

For all the disagreements, Slutsky’s original insight about the snowballing effect of random causes remains at the core of ongoing research on business cycles. A gifted statistician and frustrated economist, Slutsky revealed hidden rhythms in simulated time-series—rhythms that appear to pulse through the economy as well, often with far-reaching consequences. “The economics keep changing,” observed Lucas, “but the basic idea that we’re going to model the economy as a system of equations subject to external shocks—that’s stayed with us.”

Maxim Troshkin, a research analyst at the Minneapolis Fed, assisted in translating and interpreting the Russian documents referenced in this article.

Endnotes

1 Slutsky, Eugen. 1927. “The Summation of Random Causes as a Source of Cyclic Processes.” Problems of Economic Conditions 3 (1). Moscow: Conjuncture Institute. Ten years later, Econometrica translated the original Russian article into English; the 1937 version was revised by Slutsky and incorporated several new results (see note 5).

2 Frisch, Ragnar. 1933. “Propagation Problems and Impulse Problems in Dynamic Economics.” In Economic Essays in Honor of Gustav Cassell. London: Allen and Unwin. Reprinted in Robert Gordon and Lawrence Klein, eds. 1965. Readings in Business Cycles. Homewood, Ill.: Richard D. Irwin, p. 178.

3 Slutsky, Eugen. “Autobiography.” In Sheynin, Oscar, trans., Probability and Statistics: Russian Papers of the Soviet Period. Berlin, 2004, p. 91.

4 Chetverikov, N.S. 1959. “The Life and Scientific Work of Slutsky.” In Sheynin, Oscar, trans., Probability and Statistics: Russian Papers of the Soviet Period. Berlin, 2004, p. 100. Online (see note 3).

5 Slutsky, Eugen. 1937. “The Summation of Random Causes as a Source of Cyclic Processes.” Econometrica 5 (April), p. 110.

6 Kuznets, Simon. 1929. “Random Events and Cyclical Oscillations.” Journal of the American Statistical Association 24 (September), p. 274.

7 Frisch, ibid.

8 Lucas, Robert E. Jr. 1977. “Understanding Business Cycles.” In Karl Brunner and Allan H. Meltzer, eds., Stabilization of the Domestic and International Economy. Carnegie Rochester Series on Public Policy. Amsterdam: North Holland, pp. 7–29. Reprinted in Lucas, Robert E. Jr., Studies in Business Cycle Theory. Cambridge, Mass.: MIT Press, 1981, p. 217.

9 McGrattan, Ellen R. 1994. “The Macroeconomic Effects of Distortionary Taxation.” Journal of Monetary Economics 33, pp. 573–601; also McGrattan, Ellen R. 2009. “Capital Taxation during the Great Depression.” Working Paper 670, Federal Reserve Bank of Minneapolis.

10 Summers, Lawrence H. 1986. “Some Skeptical Observations on Real Business Cycle Theory.” Federal Reserve Bank of Minneapolis Quarterly Review 10 (Fall), p. 24.

11 Prescott, Edward C. 1986. “Response to a Skeptic.” Federal Reserve Bank of Minneapolis Quarterly Review 10 (Fall), p. 28.